Skip to content Skip to sidebar Skip to footer
Home / Prove a Periodic Function is Uniformly Continuous

Prove a Periodic Function is Uniformly Continuous

Post a Comment

Prove that $\ln x$ is not uniformly continuous on $(0,1]$

Solution 1

Is obvious right away that $\ln x$ is not uniformly continous on $\left(0,1\right]$ because it has no limit as $x\to 0$, and it is known that any uniformly continous function on a bounded interval has limits as $x$ gets close to one of the extremities.

To prove it directly, use the definition of a uniformly continous function:

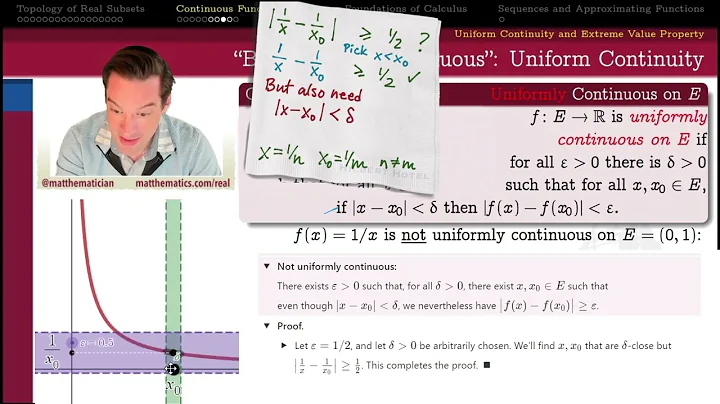
$f$ is uniformly continous when, given any $\varepsilon >0$, there exists $\delta>0$ such that, for all $x$ and $y$ in $\left(0,1\right]$: $$\text{if $x$ and $y$ are such that } \left|x-y\right|<\delta \text{, then } \left|f\left(x\right)-f\left(y\right)\right|<\varepsilon \text{ .}$$

Well, let $\varepsilon$ be a positive real number, and suppose that there existed $\delta$ satisfying the above condition. Take any $x\in\left(0,\delta\right]$. Then, for any $y$ in $\left(0,x+\delta\right)$ we would have $\left|\ln x - \ln y\right|<\varepsilon$, which means that $\ln y \in \left(\ln x - \varepsilon, \ln x + \varepsilon\right)$, and in particular, $\forall y\in \left(0,x+\delta\right), \ln y > \ln x - \varepsilon$. Well, that's clearly an absurd, because $\ln y$ tends to $-\infty$ as $y\to 0$. Thus $\ln$ cannot be uniformly continous in $\left(0,1\right]$

Solution 2

A function is not uniformly continuous if there exist two sequences $x_k, y_k$ such that $$ x_k - y_k \to 0 \qquad\text{but not}\qquad f(x_k)-f(y_k) \to 0. $$ This can be obtained directly by negating the definition of uniform continuity.

So if $\lim_{x\to x_0} f(x) = -\infty$ then such sequences can be found (take any sequence $x_k\to x_0$ and a sequence $y_k \to x_0$ such that $|f(y_k)-f(x_k)|\ge 1$) and the function is not uniformly continuous.

Related videos on Youtube

Comments

  • Prove that $\ln x$ is not uniformly continuous on $(0,1]$.

    Got all confused on how to prove this kind of things... Any hints would be great!

  • It would be more direct to use $\ln(\delta)-\ln(\delta/2)=\ln 2$.

  • Yes, it would. However, I think it's better to have an answer that uses only the fact that that the function is unbounded, to show that this is not a problem with functions with the $f(ab)=f(a)+f(b)$ property, but a more general problem.

  • take $x_{k}= e^{-k}$ and $y_{k}= e^{-(k+1)}$ for instance.

  • Why do you use the interval (0,δ], instead of (0,1]? @fonini

  • I want to show that $f$ doesn't satisfy the quoted property in the yellow box. That property says that: "for any epsilon, there exists a delta such that blablabla". This means that I want to show that there is a special epsilon such that "blablabla" will never happen, no matter what delta I choose. Well, I have found this special epsilon (actually, I claim that any positive epsilon is enough). Now, I need to show that there is no delta such that "blablabla" happens. I do it by supposing that there is such a delta, and then I arrive at an absurd.

  • How do I arrive at the absurd? The property "blablabla" says that, for any $x,y\in(0,1]$, "blublublu" happens. What I do is just showing that this is false, because is may be alright for $x> \delta$, but the special cases in which $x\leq\delta$ lead to the absurd. Let me know if I wasn't clear @Annie

Recents

Related

Source: https://9to5science.com/prove-that-ln-x-is-not-uniformly-continuous-on-0-1

daddariochimand.blogspot.com

Source: https://hamiltonlosione90.blogspot.com/2022/10/how-to-prove-that-function-is-not.html

Post a Comment for "Prove a Periodic Function is Uniformly Continuous"