Mcat Bernoulli s Equation Continuity Equation Ormula Bernoulli Specific Gravity

Learn key MCAT concepts about fluid mechanics, plus practice questions and answers

(NOTE: THIS GUIDE IS PART OF OUR MCAT PHYSICS SERIES.)

Table of Contents

Part 1: Introduction to fluids

Part 2: Definition and applications

Part 3: Density, buoyancy, and applications of pressure

a) Density and specific gravity

b) Buoyancy

c) Hydrostatic pressure

d) Pressure and depth

Part 4: Fundamental laws

a) Pascal's law

b) Poiseuille flow

c) Continuity equation

d) Bernoulli's equation

Part 5: High-yield terms and equations

Part 6: Passage-based questions and answers

Part 7: Standalone questions and answers

Part 1: Introduction to fluids

As a study of substances including liquids, gases, and plasmas, the study of fluids is extremely important for a lot of real-world applications of physics, such as blood flow in the circulatory system.

This guide won't necessarily follow a self-contained narrative from start to finish. Instead, we'll start with a basic definition of fluids, which we'll then combine with other physics intuitions to think about other implications. This unit should give you a comprehensive understanding of the fluid-related concepts you'll see on the MCAT, as well as showing how concepts from other topics have implications everywhere!

On the MCAT, fluid mechanics is a medium-yield topic. Below, the most important terms are in bold font. When you see one, try to define it in your own words, and use it to create your own examples. This is a great way to check your understanding, and phrasing things in a way that makes the most sense to you will make studying much easier (and much more effective!) in the long run.

Part 2: Definition and applications

What is a fluid? You might know that fluids don't only refer to liquids—other states of matter, like gases, are fluids as well. Let's try to find a better definition of what a fluid is.

A fluid is a substance that will move under any shear stress. A shear stress is the result of a force that acts on the material from away from its center of mass.

When we place a fluid into a container (like water into a cup), the shear forces will continue to act on the fluid and shape it until it reaches an equilibrium.

Figure: The liquid doesn't settle until its surface is flat, because there is no longer a horizontal component of gravity exerting a shear stress here.

We can classify the flow of fluids as laminar or turbulent. Think of the difference between a calm stream and a river with rapids. The former is slow and smooth; the latter is fast-moving and full of violent disturbances. This is generally the case with slow-moving and fast-moving fluids. Low-velocity fluids create laminar flow, and high-velocity fluids create turbulent flow.

In laminar flow, a slight disturbance is quickly corrected in an orderly manner, and in turbulent flow, a disturbance forms eddies and causes a much greater interruption.

Figure: Laminar (left) vs. Turbulent (right) flow caused by a circular disturbance.

You may also have noted the distinct spherical shapes that water droplets form, or the ability of water to "creep" up sheets of paper. Surface tension is a result of cohesion between the same type of molecules on the surface of the fluid. It allows the surface of a liquid to support light things, such as a leaf or an insect, which would otherwise sink.

Surface tension is also the reason water droplets form on a surface rather than covering the entire surface with an infinitesimal layer of liquid.

Figure: Among other effects, surface tension allows for liquid droplets to form.

A similar effect occurs in capillary action, when a fluid appears to "creep up" a solid material and seemingly defy gravity. This effect occurs when molecules in the fluid are more attracted to the solid material than other molecules in the fluid. This attraction between different molecules is called adhesion.

 Part 3: Density, buoyancy, and applications of pressure

a) Density and specific gravity

The behavior of fluids depends partially on its density. Density is a measure of how much mass a given fluid has per unit of volume. The equation is pretty simple:

$$\rho = \frac{m}{v} $$ $$\mbox{where }\rho \mbox{ = density,}$$ $$m \mbox{ = mass,}$$ $$V \mbox{ = volume}$$

The density of water is 1 kg/L (or 1 g/mL or 1 g/cm³). Convenient!

When different fluids interact, their relative densities greatly influence what happens. The density of water sets a baseline, and the density of something compared to water is called its specific gravity.

$$SG = \frac{\rho}{\rho_{water}} $$ $$\mbox{where }\rho \mbox{ = density of the substance,}$$ $$\rho_{water} \mbox{ = density of water}$$

b) Buoyancy

The buoyancy of a floating object is the force that opposes gravity and pushes the object upwards.

Figure: A free-body diagram of an object suspended in a fluid. Buoyancy (FB) opposes gravity (Fg).

We can calculate the buoyancy force on an object suspended (at rest) in a fluid thanks to Archimedes' principle. As the story goes, Archimedes jumped out of his tub and shouted, "Eureka!" when he realized that when an object is suspended at rest in a fluid, the buoyant force on an object is equal to the weight of the fluid displaced by the object. In equation form, this means:

$$F_B = m_{\mbox{displaced}}\times g = \rho_{\mbox{fluid}}\times V_{\mbox{object}} \times g$$ $$\mbox{where }F_B \mbox{ = buoyant force,}$$ $$m_{displaced} \mbox{ = mass of the displaced fluid,}$$ $$\rho_{fluid} \mbox{ = density of the fluid,}$$ $$V \mbox{ = volume of the submerged object,}$$ $$g = 9.8\space {}^m/_{s^2}$$

Remember that by definition, the "weight" of an object is equal to its mass (m) times the acceleration due to gravity (g).

There are a few things to note about those equations. First, in the rightmost equation, we used the definition of density (ρ=m/V) to substitute ρ*V for m.

Second, volume V is the volume of the object. We say this to make another implication of Archimedes' principle clear: the volume of the submerged object and of the displaced liquid are always equal; the equal weight occurs only when the object is in equilibrium, or at rest. When the object is at rest, it must have the same density as the fluid around it, meaning the same volume will have the same weight. If the object does not have the same density as the fluid around it, it will change its position within the fluid accordingly:

• Objects will rise when their weight is less than the buoyant force, or when they are less dense than the surrounding fluid.

• Objects will sink when their weight is more than the buoyant force, or when the object is denser than the surrounding fluid.

• Objects will float at the height in the liquid where their weight equals the buoyant force, or when they have the same density as the surrounding fluid.

Remember also that the volume of the displaced liquid is not necessarily equal to the volume of the object. This is because objects can be partially submerged, or float on top of the fluid.

Figure: A rubber ducky will float in water. Part of it is submerged, and part of it remains above the surface of the water. The volume of the displaced liquid is noted by the shaded portion of the duck.

Luckily, the specific gravity will also tell you how much of a floating object (with uniform density) will be submerged in water. For instance, a specific gravity of 0.7 indicates that 70% of the object will be submerged, while 30% of the object remains above the level of the water.

c) Hydrostatic pressure

Objects suspended in fluids not only feel pressure from below, but also from all the fluid that pushes down on them from above. Hydrostatic pressure refers to all of the different stresses on an object at rest in a fluid: whether in air on the surface of the Earth or swimming underwater.

d) Pressure and depth

If you ever take a flight somewhere or go scuba diving, you'll know the significance of pressure as a function of depth. When you fly thirty thousand feet in the air, or dive even a few dozen feet underwater, your ears will probably pop due to the change in pressure. The following equation represents this phenomenon in a fluid:

$$P = \rho \times g \times h$$ $$\mbox{where }P \mbox{ = pressure,}$$ $$\rho \mbox{ = density of the fluid,}$$ $$g = 9.8\space {}^m/_{s^2},$$ $$h\mbox{ = depth below the surface}$$

This equation is for the gauge pressure, because it ignores atmospheric pressure; notice that the pressure at the surface is zero because h=0. Gauge pressure is still useful when we are only dealing with objects submerged in fluids, but if we want absolute pressure, we have to add atmospheric pressure (atm) to it.

Devices called barometers are used to measure pressure. Barometers are filled with a liquid of a known density and are open at one end. Since the pressure exerted by the liquid must be equal to the atmospheric pressure at the open end, the height of the fluid column can be used to determine the local atmospheric pressure.

In a simple mercury barometer, the fluid used is mercury. The height of the mercury column can be used to determine the pressure of the air around the barometer. For a standard barometer, a height of 760 millimeters of mercury (mmHg) corresponds to 1 atm. This is also equal to 101,325 Pascals (Pa).

Part 4: Fundamental laws

a) Pascal's law

Pascal's law states that pressure applied to a liquid is transmitted evenly across all points at the same height h.

This principle is well-utilized in hydraulic presses. If you have a water-filled pipe with differently sized openings, the pressure input on the small opening will be the same as the pressure output from the larger one. Since force equals pressure times area, the large opening will output a force much larger than the one you put in on the small end. This allows mechanics, for example, to lift heavy cars very efficiently with simple machinery.

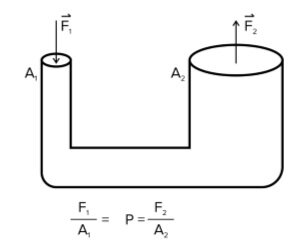
Figure: Force 2 is much larger than Force 1. P is constant throughout.

Note that the work done, the force over a distance, is also the same on each side. This means that even though it requires a smaller force, you have to push the water down farther on the small side to get a smaller lift on the large side.

b) Poiseuille flow

Viscosity is a measure of how much resistance a fluid has against stresses. You can think of it as the "internal friction" of a fluid, or as the "thickness" of it. Fluids that have high viscosity flow more slowly, have more internal friction, and are "thicker".

Poiseuille flow describes the flow of viscous fluids in a circular pipe. It's important to remember that although the fluid flowing through the pipe may have a certain average velocity, the fluid flow will not have the same velocity at all points in the cross-section of the pipe!

Since there is more interaction between the fluid and the walls, the fluid at the walls of the pipe will move a little slower than the fluid in the center of the pipe. In certain conditions, this flow can be described by Poiseuille's law:

$$Q = \frac{\pi r^4\Delta P}{8\eta L}$$ $$\mbox{where }Q \mbox{ = flow rate,}$$ $$\pi \mbox{ = 3.14,}$$ $$r\mbox{ = radius of the pipe}$$ $$\Delta P\mbox{ = rate of pressure drop,}$$ $$\eta \mbox{ = viscosity of the fluid}$$ $$L \mbox{ = length of the pipe}$$

Figure: Poiseuille flow.

The MCAT will often test your knowledge of Poiseuille flow in the context of blood vessels, which are modelled as circular pipes. Notice that flow will increase with the fourth power of the radius of the pipe or blood vessel, and will decrease inversely with the length of the pipe or blood vessel.

c) Continuity equation

When a fluid is flowing, it must also follow the law of conservation of mass. When a fluid is incompressible (for instance, a liquid) we can assume that the volume flow rate of a fluid is constant. In equation form, this means:

$$\frac{\Delta V}{\Delta t} = constant$$ $$\mbox{where }V \mbox{ = volume,}$$ $$t \mbox{ = time}$$

Knowing that volume equals area times length and that length per time is velocity, we can also say that:

$$A\times v = constant$$ $$\mbox{where }A \mbox{ = the area of the pipe,}$$ $$v \mbox{ = linear flow rate}$$

We can use this to our advantage whenever we come across pipes of different sizes.

d) Bernoulli's equation

Think back to kinematics, and when we studied the conservation of energy. What did that state?

Well, we know that the sum of kinetic energy and potential energy must be constant throughout a system.Bernoulli's equation is a form of this conservation of energy equation. It states that the sum of the pressure energy (a form of internal energy), kinetic energy per volume, and potential energy per unit volume remains constant:

$$P + \frac{1}{2}\rho v^2 + \rho gh=\mbox{constant}$$ $$\mbox{where }P \mbox{ = pressure energy,}$$ $$\frac{1}{2} \rho v^2\mbox{ = kinetic energy per unit volume,}$$ $$\rho gh \mbox{ = potential energy per unit volume}$$

The Venturi effect is a result of Bernoulli's equation. When a fluid passes through a constriction in a pipe, the fluid's velocity increases and pressure decreases.

A Pitot tube is a flow measuring device. It measures the speed of flow indirectly by directing into the tube, which contains a fluid. The pressure on this fluid can be used with Bernoulli's equation to solve for the flow speed.

Part 5: High-yield terms

Fluid: a substance that will flow under any shear stress; includes liquids, gases, and plasmas
Shear stress: an external force that acts coplanar to the material's cross-section
Density: an object's mass per unit volume
Specific gravity: the ratio of a fluid's density to that of water
Buoyancy: the force on a floating object that opposes gravity
Archimedes' principle: the buoyant force on a suspended object is equal to the weight of the fluid it displaces
Hydrostatic pressure: pressure resulting from all stresses on an object at rest in a fluid
Gauge pressure: the pressure difference between the surface of a fluid and a submerged point
Absolute pressure: gauge pressure plus the atmospheric pressure
Atmospheric pressure: pressure due to Earth's atmosphere; 1 atm = 101,000 Pa = 760 mmHg
Pascal's law: pressure applied to a liquid is distributed evenly across the liquid
Work: energy transferred by a force applied over a distance
Viscosity: a measure of the resistance a fluid has against stresses; a measure of the "internal friction" or "thickness" of a fluid
Poiseuille flow: the flow of a viscous fluid in a pipe whose front takes on a parabolic shapeIncompressibility: an approximation often used for fluids; implies a constant volume flow rate
Laminar flow: the smooth flow caused by low-velocity moving fluids
Turbulent flow: the chaotic flow characterized by eddies caused by high-velocity moving fluids
Surface tension: the result of strong surface bonds that may allow liquids to form droplets or hold light objects
Dynamic pressure: the pressure from moving fluids, or the kinetic energy per unit volume
Bernoulli's equation: a form of energy conservation that conserves the sum of pressure energy, kinetic energy per unit volume, and potential energy per unit volume
Venturi effect: describes a fluid's increase in velocity and decrease in pressure when it enters a constriction
Pitot tube: a device that measures flow velocity by monitoring pressure on a fluid in a tube

Density $$\rho = \frac{m}{V}$$ Specific Gravity $$ SG=\frac{\rho}{\rho_{\mbox{water}}}$$ Buoyant force $$F_B = m_{\mbox{displaced}}\times g = \rho_{\mbox{fluid}}\times V_{\mbox{object}} \times g$$ Hydrostatic pressure $$P = \rho \times g \times h$$ Atmospheric pressure $$\mbox{1 atm = 101,000 Pa}$$ Pascal's law $$\frac{F_1}{A_1}=\frac{F_2}{A_2}=\mbox{constant}$$ Continuity equation $$\frac{\Delta V}{\Delta t} = \mbox{constant} = A\times v$$ Dynamic pressure $$q = \frac{1}{2}\times \rho \times v^2$$ Bernoulli's equation $$P + \frac{1}{2}\rho v^2 + \rho gh=\mbox{constant}$$

_{Acknowledgements: Brian Dolan}

Part 6: Passage-based questions and answers

A team of public health experts and engineers is overseeing the construction of a pipeline to bring safe drinking water to a community. The water source contains harmful compounds, and the resulting water must be treated. One of the final stages of water treatment is disinfection, in which a disinfectant, such as chloramine, is added to kill any microbes surviving previous stages of treatment.

To continually inject disinfectant to the moving water supply, the team has opted to employ a Venturi siphon, a device that utilizes the Venturi effect and local points of low pressure to draw fluid from a reservoir, in this case, a reservoir filled with disinfectant, into the main pipeline.

The shape of the pipe where the siphon is located is shown in Figure 1. At point 1, the radius is 0.5 m, the absolute pressure is 200,000 Pa, and the linear flow velocity is 0.5 m/s. The radii at points 3 and 4 are 0.25m and 1m, respectively.

Figure 1: The geometry of the pipe where the siphon will be placed.

The water is assumed to be incompressible and flowing without turbulence.

Question 1: At which point in the pipe is the water flowing the fastest?

A) Point 1, because the flow is closest to the source

B) Point 2, because the flow is being constricted gradually

C) Point 3, because it has the smallest radius

D) Point 4, because the largest radius offers the least resistance

Question 2: In the diagram above, the siphon itself is not shown. At which point should the siphon be placed if it is to draw disinfectant from the reservoir?

A) Point 1, the flow has not yet been disturbed

B) Point 2, because it has the lowest pressure

C) Point 3, because it has the lowest pressure

D) Point 4, because it has the most free space

Question 3: What is the linear flow velocity of the water at point 4?

A) 0.1 m/s

B) 0.125 m/s

C) 0.25 m/s

D) 0.75 m/s

Question 4: What could be the gauge pressure at point 3?

A) 200,000 Pa

B) 99,000 Pa

C) 210,000 Pa

D) 90,000 Pa

Question 5: Imagine the tube in the image becomes clogged at point 4. Assuming a required force of 100N to unclog it, what is the minimum force required at point 1?

A) 25 N

B) 50 N

C) 75 N

D) 100 N

Answer key for passage-based practice questions

1. Answer choice C is correct. The Venturi effect states that a fluid increases in speed and decreases in pressure when it enters a constriction. Since point 3 has the smallest radius and is the greatest constriction, the flow is fastest there (choice C is correct). Proximity to the source does not affect the flow rate (choice A is incorrect); the flow rate depends on the magnitude of the constriction, not the rate of constriction (choice B is incorrect); and the appeal to circuit-like resistance does not hold here because water is not compressible, and its volume flow rate must be conserved (choice D is incorrect).

2. Answer choice C is correct. A localized occurrence of low pressure is needed to draw disinfectant from the reservoir. The Venturi effect relates low pressure with linear flow rate. When a fluid passes through a constriction in a pipe, the fluid's velocity increases and pressure decreases. As a result, point 3 must have the lowest pressure (choice C is correct). There is no turbulence (choice A is not correct); point 2 does not have the lowest pressure (choice B is not correct); and free space does not create any suction (choice D is not correct).

3. Answer choice B is correct. The fluid can be approximated as incompressible, so the volume flow rate (∆V/∆t) is constant. Volume = Area*length, so:

$$\frac{\Delta V}{\Delta t} = \frac{\Delta (A\times l)}{\Delta t} = A\times v$$

The area of a circular cross section is πr², the radius at point 1 is 0.5m and the linear flow rate is 0.5m/s, so the volume flow rate at point 1 is π*(0.5)²*0.5. Set this equal to π*1²*v, the volume flow rate at point 4. Solving for v gives v=0.125 (choice B is correct).

4. Answer choice D is correct. Gauge pressure is equal to the absolute pressure minus the atmospheric pressure, so gauge pressure at point 1 can be calculated as 200,000-101,000=99,000 Pa. Additionally, due to the Venturi effect, the pressure at point 3 has to be lower than the pressure at point 1. The only option that satisfies this condition is D, 90,000 Pa (choice D is correct).

5. Answer choice A is correct. Pascal's law states that an applied pressure is distributed evenly across an incompressible liquid. Applied pressure is equal to force divided by area, and we know both the cross-sectional areas at points 1 and 4 and also the required force at point 4.

Thus: F/π(.5)2=100/π(1)2. Solving for F gives F=100*0.25=25N (choice A is correct).

-----

Part 7: Standalone questions and answers

Question 1: How does doubling the depth of measurement affect the absolute pressure?

A) Absolute pressure increases by a factor of 2

B) Absolute pressure increases by a factor of less than 2

C) Absolute pressure increases by a factor of more than 2

D) Absolute pressure does not change with depth

Question 2: Which of the following is the best representation of Archimedes' principle?

A) A heavier person making a bigger splash when jumping into a pool

B) A feather floating on the surface of water

C) A droplet of water retaining its shape on a flat surface

D) A person causing a raft to sink deeper by stepping into it

Question 3: If the pipe openings of a hydraulic lift have areas of 1m2 and 10m2, how far must the liquid on a smaller-diameter end be displaced to raise an object on the larger-diameter end 0.5 meters in the air?

A) 0.5m

B) 2.5m

C) 5.0m

D) 7.5m

Question 4: Water and oil do not mix due to differing polarities. If they are in the same container, a layer of oil will sit on top of a layer of water. What does this imply about the specific gravity of oil?

A) The specific gravity of oil is less than 1

B) The specific gravity of oil equals 1

C) The specific gravity of oil is greater than 1

D) There is not enough information to conclude anything

Question 5: A 0.1m metal cube floats between layers of oil and water. The top 0.07m of the cube is in the oil, while the bottom 0.03m of the cube is in the water. If the densities of water and oil are 1000kg/m3 and 700kg/m3, respectively, what is the weight of the cube?

A) 5 N

B) 6 N

C) 7 N

D) 8 N

Answer key for standalone practice questions

1. Answer choice B is correct. Absolute pressure is the sum of gauge pressure and atmospheric pressure.

$$P_1 = P_{gauge}+P_{atm}$$

Gauge pressure is directly proportional to depth, but atmospheric pressure doesn't depend on depth underwater. Doubling depth gives:

$$P_2 = 2P_{gauge}+P_{atm}$$

which is less than

$$2P_1 = 2P_{gauge}+2P_{atm}$$

So, doubling depth increases absolute pressure, but by a factor of less than 2 (choice B is correct).

2. Answer choice D is correct. Archimedes' principle states that a submerged object will displace its volume in water, and if it is at rest, it will displace its own weight. Option D represents a raft becoming heavier and then sinking lower, displacing more water (choice D is correct). Splash size depends on more than just the weight of the object creating the splash (choice A is incorrect), and a feather floating on water and a droplet retaining its shape both represent effects of surface tension (choices B and C are incorrect).

3. Answer choice C is correct. Recall that energy has to be conserved in a hydraulic lift, so the work done on one end is the same as the work done by the other end. Work equals force times distance:

$$W=F\times d$$ $$W_1 = F_1 \times d_1 = F_2\times d_2 = W_2$$

Pascal's law tells us that the Force per unit area is the same on each end,

$$\frac{F_1}{A_1} = \frac{F_2}{A_2}$$

A₂=10A₁, so we can use Pascal's law to say that F₂=10F₁. Plugging these into the work equation gives F₁*d₁=10F₁*d₂. F₁ cancels from both sides and d₁=10*d₂=5m (choice C is correct).

4. Answer choice A is correct. Specific gravity is the ratio of a fluid's density to the density of water. Since oil sits on top of water, it must be less dense. Since it is less dense than water, the ratio of its density to that of water is less than 1 (choice A is correct).

5. Answer choice D is correct. Archimedes' principle tells us that the cube must displace its weight in each of the fluids.

Since 0.1 x 0.1 x 0.03 m³ of its volume is in the water, the weight of that portion equals 1000 kg/m³ x (0.1 x 0.1 x 0.03 m³)x g=0.3 x g Newtons. Since 0.1 x 0.1 x 0.07 m³ of its volume is in the oil, the weight of that portion equals 700 kg/m³ x (0.1 x 0.1 x 0.07 m³)x g=0.49 x g Newtons. Adding the two and approximating g=10m/s² gives .79 x 10=7.9 N, which is rounded up to 8N (choice D is correct).

daddariochimand.blogspot.com

Source: https://www.shemmassianconsulting.com/blog/fluid-mechanics-mcat